Bar Models to solve KS2 word problems

Bar Models are great for solving multi-step word problems and the KS2 SATs problems that many pupils will face this week are no different.

As teachers and pupils get ready for their end of KS2 exams, we waited until now to release this blog post so that teachers don’t use the bar model approach in the last week as a way of cramming in support for their pupils. Many teachers and pupils will feel the strain of the two maths reasoning papers, which a large portion of the country struggled on last academic year. In fact, we are hearing that some schools have even started Saturday classes to support their pupils, which highlights the pressure many of us are under.

We’ve seen many more multi-step word problems appearing in SATs preparation questions and inevitably get very excited about showcasing a few using bar models. With the focus now much more on problem-solving skills.

Question 1

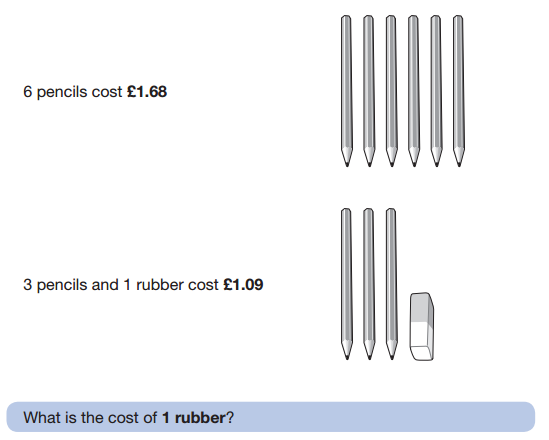
First up is the above question. This is a good example of a two step problem and we can use the part-whole model for both steps. The first representation is of the 6 pencils which altogether costs £1.68. We can represent them using a consistent bar. This bar model is a part-whole model as there are 6 “parts” within one “whole” bar. We can see that each part is equal as they are all the same type of pencils, therefore we can work out the cost of each pencil. £1.68 ÷ 6 = £0.28. Each pencil is 28p, which leads us to the second model we can draw…

We know each pencil is now 28p and hence the cost of three pencils is 3 X 0.28 = £0.84. This can be added to the model…

Finally, We know that altogether 3 pencils and a rubber costs £1.09 and the cost of three pencils is £0.84 and therefore we can work out that the rubber must be £1.09 – £0.84 = £0.25. A rubber must cost 25p.

Some pupils may not need the bar model approach for this question and can work systematically through the question to get the exact same answer. The bar model approach, in this case, help us to visualise the problem, break down the question and make those steps especially if you typically find these questions more challenging.

Question 2

Our second example is a very abstract question, that came up in the last year’s SATs paper was the below equation.

2x + 4 = 100

We’ve been delivering more sessions on how to use bar models in algebra lately and this is one of those questions where bar models really do help in understanding and solving algebraic equations like this. How would the model look? Something like this…

If 2 lot’s of a number (X) + 4 gives us a total of 100, what can you say about the two numbers?

Right, 2X must be equal to 100-4 = 96. What would x equal?

If 2X=96, then X must be 96 ÷ 2 = 48. X = 48. Can we check our answer?

Using Bar Models in KS2 SAT’s

The above two examples are a good way to illustrate problem-solving questions, including algebraic questions which seem challenging at first but when drawn out, are simple cases where the core always comes back to using the four operations. Bar Models are a must have resource in every classroom at Primary level and can also be used in many topics in Secondary Maths too.