Bar Model Questions to Introduce to your class

We’ve decided to suggest some bar model questions which schools can use to start the process of using Bar Models in each year group. If you have always wanted to use bar models but weren’t sure what question to start off with, then the questions below will help you to start using bar modelling in your class.

Year 1/2 Bar Model Questions

The first model that we must master is called the part-whole model. At year 1/2 level it will be a very basic question and we recommend using numbers within 20 to start off with. Something like,

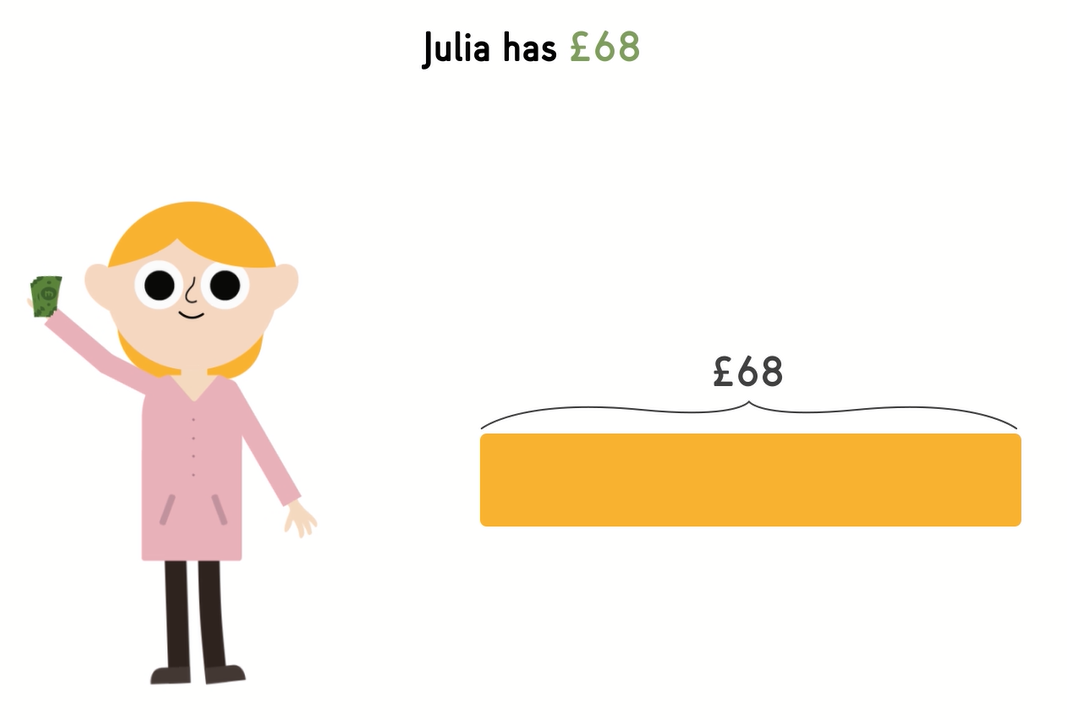
“Sam has 10 stickers and James has 5 stickers. How many stickers do they have altogether?”

The part-whole bar model is one where there is only one bar and within the bar, we have different parts. All the parts make the whole bar and hence the name part-whole model. The part-whole model is the basis of all bar models, including the comparison model and other derivatives such as the before and after situation models. This is why it’s imperative that we go through each of the 5 standard part-whole model scenarios with our class and when going through questions representing each scenario, we assess pupils understanding of each question and whilst doing this we also need to assess whether our pupils understand the relevance of each part of the model. Let’s look at an example question:

When considering setting questions for your class, consider questions where the following are given and pupils are asked to work out the (?):

1. part 1 (given) + part 2 (given) = whole (?)

2. whole (given) – part 1 (given) = part 2 (?)

3. whole (given) – part 2 (given) = part 1 (?)

This part-whole situation is covered in most Singapore Maths books in Year 1 and initially looks like a number split into two numbers like the number 6 below. You would split the number in a way that allows you to complete a calculation in the most efficient way.

If we focus on just the partitioning of 6, we call this the part-part-whole diagram and also the number bond diagram. In essence, the diagram shows what is happening in the part-whole model in the video. For this reason, the part-part-whole diagram and the use of ten-frames (a CPD training course we offer for EYFS and Year 1) are pre-requisite of the bar model approach at KS1 level. Play around with both and then ask the above questions to your Year 1/2 class.

KS2 Bar Model Questions

The problem we have at KS2 level is that the expectations from teachers are a lot higher as they have gone through many different topics by the time pupils get to upper KS2. Why is that a problem? Well it isn’t a problem as such, having high expectations is great but as pupils have had different layers of experience in various topics, they haven’t necessarily had much or any experience in using bar modelling. This means that teachers are expecting a lot from their class but without actually going through the normal steps you would have done in KS1 and in lower KS2.

At Lower KS2 we would suggest going through part-whole models until you feel your class understand the different parts of the model. Once your pupils are showing signs that they understand what type of model you would use in a part-whole model and are confidently labelling and describing each part then the next step would be to look at comparison models. Bar Model Questions such as the one below are typically asked from Year 2 onwards.

The Liverpool-Chelsea FC question is a basic model but it allows for a lot of discussions to take place and therefore a thorough understanding of the model. In the video we have put in the question ‘what is this?’ – What does the extra part represent, is a key question we have to ask when using the comparison model.

At Upper KS2 level the part-whole model and basic comparison models are a good starting point and you would probably focus on questions which involve larger numbers, something upper KS2 pupils should be used to. This doesn’t mean we should always start off with numbers between 1million and 10 million (in year 6), in fact, we would say for new bar model concepts, stick to smaller numbers and just focus on the actual strategy and model. Once pupils understand the model better, they can then generalise and move to questions where numbers are more typical for their year groups. The concept here is key and not just drawing the model but actually understanding each part and how it relates to the question.

KS2 pupils will feel more challenged by questions like the one in this video below. What you will notice in the video below is that even in more challenging questions the part-whole model is still present somewhere…can you notice it in this video?

KS2 pupils will be able to understand the process a lot better if we, as teachers, can “convince them” to take part and have a go by using concrete materials and following the CPA approach, especially when introducing new models to the class. In our training sessions we use paper to represent the bars and this acts as the concrete representation. It can be a challenging lesson everytime you introduce/reintroduce bar models and that’s why we insist on schools getting our training, which has helped many thousands of teacher across England.

Where ever you are in your implementation of bar modelling or Singapore Maths, staff can and will find certain lessons very challenging. This is why we suggest all schools get our bar model and visualisation training, which has helped thousands of teacher across England, not only that we are giving away one year’s free subscription to our animated online videos for all schools booking at least 1 full day training with us.

Want to see a bar model question being introduced to a small group? Watch a Bar Modelling lesson

Ready to book your training? Contact us now to start you bar modelling journey with us.